Quartz Properties:
Rupture Calculations
Fused quartz stands firm at room temperature, yet when exposed to high heat, it reveals its adaptability—softening gracefully without a sharp melting point like crystalline materials. Its transition from solid to plastic-like behavior is a journey through the transformation range, where viscosity changes fluidly with temperature. This remarkable flexibility reflects the power of resilience, showing us that even the strongest can bend and adapt when faced with intense pressure, while still maintaining their core integrity.
Rupture Formula For Tubing
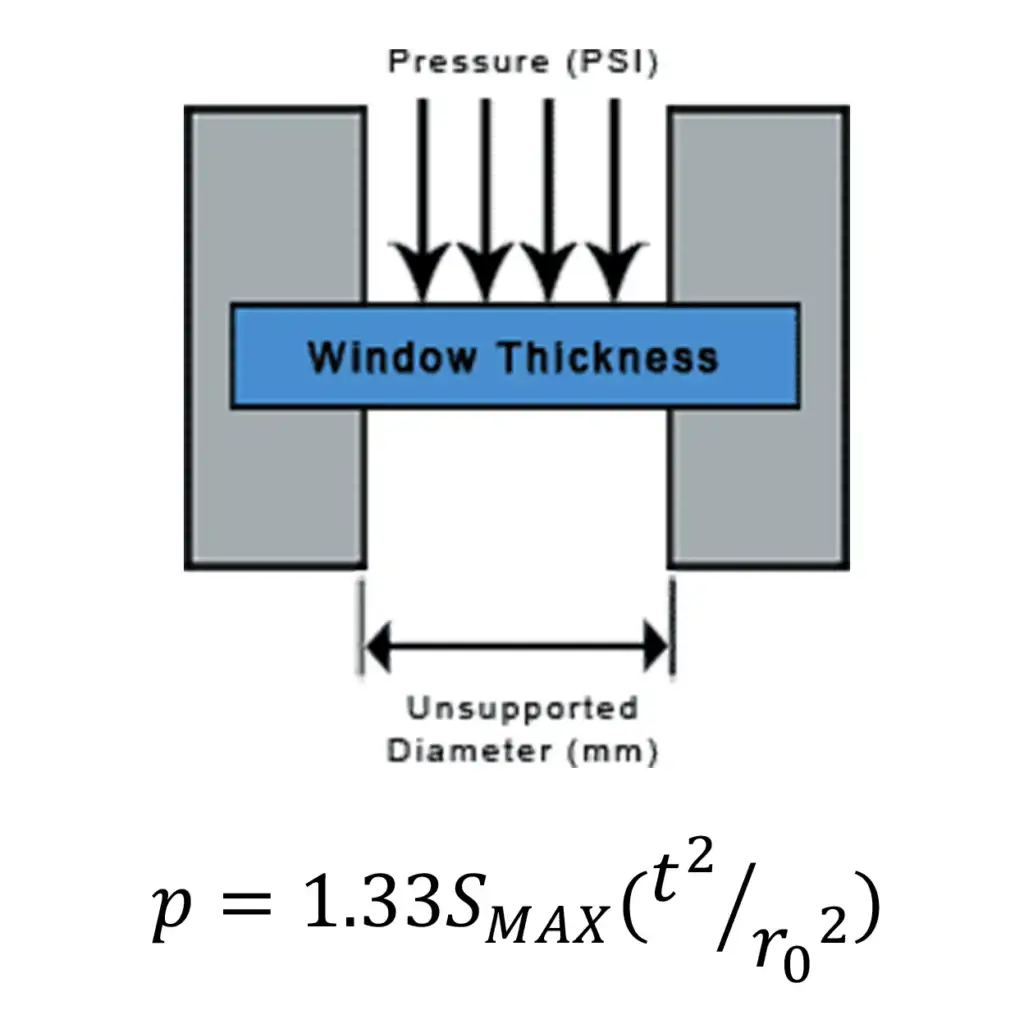
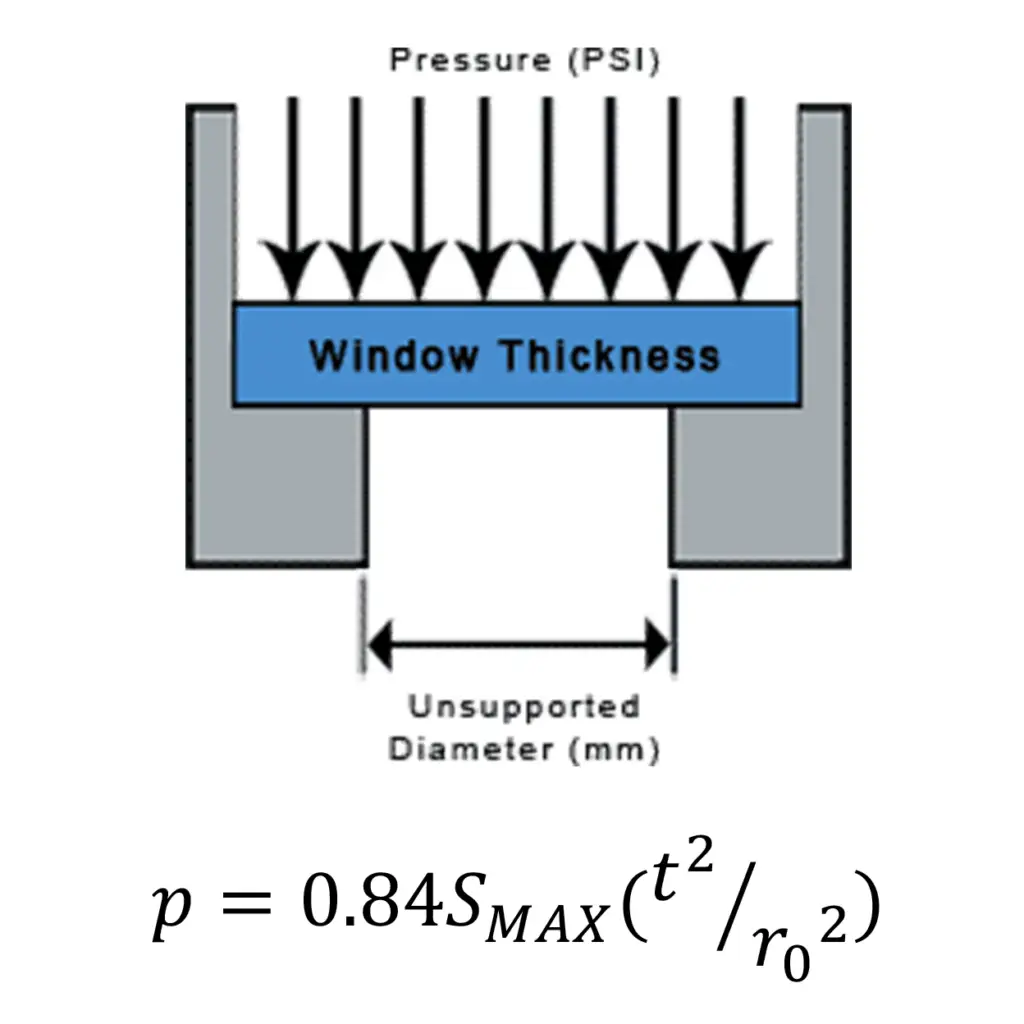
Rupture Formula For Discs And Plates
Many applications of fused quartz discs, plates and sight glasses require calculating pressure differential. The following formulae can be used for room temperature applications of circular parts with either clamped or unclamped edges.
- p = Pressure differential (Pa)
- r0 = Unsupported disc radius (mm). For plates substitute r0 with width
- SMAX = Maximum stress (approx. 7 to 1 safety factor) 7.0 x 106 Pa
- t = Disc Thickness (mm)
However, the following factors will affect the strength of these parts and must be considered when using the formula:
- Surface should be highly polished and free of scratches
- Means by which a sample is clamped into a pressure device
- The clamping material used
- The thermal gradients expected across the surface and between the surfaces
- The rate of pressure increase that will be applied
- Temperature of specimen
